NO.
|
TOPIC
|
SUBJECT CONTENT
|
|
1 |
Numbers |
·
Use natural numbers, integers (positive, negative and zero), prime
numbers, common factors and multiples, rational and irrational
numbers, real numbers;
·
Continue given number sequences, recognise patterns within and across
different sequences and generalise to simple algebraic statements
(including expressions for the nth term) relating to such
sequences. |
|
2 |
Squares, square roots, cubes and cube roots |
·
Calculate squares, square roots, cubes and cube roots of numbers |
|
3 |
Vulgar and decimal fractions and percentages |
·
Use the language and notation of simple vulgar and decimal fractions
and percentages in appropriate contexts;
·
Recognise equivalence and convert between these forms |
|
4 |
Ordering |
·
Order quantities by magnitude and demonstrate familiarity with the
symbols  . . |
|
5 |
Standard form |
·
Use the standard form  where n is a positive or negative integer, and where n is a positive or negative integer, and
 . . |
|
6 |
The
four operations |
·
Use the four operations for calculations with whole numbers, decimal
fractions and vulgar (and mixed) fractions, including correct ordering
of operations and use of brackets. |
|
7 |
Estimation |
·
Make estimates of numbers, quantities and lengths;
·
Give approximation to specified numbers of significant figures and
decimal places;
·
Round off numbers to reasonable accuracy in the context of a given
problem. |
|
8 |
Ratio, proportion, rate |
·
Demonstrate an understanding of the elementary ideas and notation of
ratio, direct and inverse proportion and common measures of rate;
·
Divide a quantity in a given ratio;
·
Use scales in a practical situation;
·
Calculate average speed;
·
Express direct and inverse variation in algebraic terms and use this
form of expression to find unknown quantities. |
|
9 |
Percentages |
·
Calculate a given percentage of a quantity;
·
Express one quantity as a percentage of another;
·
Calculate percentage increase or decrease;
·
Carry out calculations involving reverse percentages, e.g. finding the
cost price given the selling price and the percentage profit |
|
10 |
Use
of a scientific calculator |
·
Use a scientific calculator efficiently;
·
Apply appropriate checks of accuracy. |
|
11 |
Everyday mathematics |
·
Use directed numbers in practical situations (e.g. temperature change,
tide levels);
·
Use current units of mass, length, area, volume, capacity and time in
practical situations (including expressing quantities in terms of
lager or smaller units);
·
Calculate times in terms of 12-hour and 24-hour clock (including
reading of clocks, dials and timetables);
·
Solve problems involving money and convert from one currency to
another;
·
Use given data to solve problems on personal household finance
involving earnings, simple interest, and compound interest (without
the use of formula), discount, profit and loss.
·
Extract data from tables and charts. |
|
12 |
Graphs in practical situations |
·
Interpret and use graphs in practical situations including travel
graphs and conversion graphs;
·
Draw graphs from given data;
·
Apply the idea of rate of change to easy kinematics involving
distance-time and speed-time graphs, acceleration and retardation;
·
Calculate distance travelled as area under a linear speed-time graph. |
|
13 |
Graphs of functions |
·
Construct tables of values and draw graphs for functions of the form
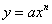 where n = -2, -1, 0, 1, 2, 3, and simple
sums of not more then three of these and for functions of the form where n = -2, -1, 0, 1, 2, 3, and simple
sums of not more then three of these and for functions of the form
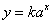 where a is a positive integer; where a is a positive integer;
·
Interpret graphs of linear, quadratic, reciprocal and exponential
functions;
·
Find the gradient of a straight line graph;
·
Solve equations approximately by graphical methods;
·
Estimate gradients of curves by drawing tangents. |
|
14 |
Coordinate geometry |
·
Demonstrate familiarity with Cartesian coordinates in two dimensions;
·
Calculate the gradient of a straight line from the coordinates of two
points on it;
·
Interpret and obtain the equation of a straight line in the form of
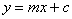 ; ;
·
Calculate the length and coordinates of the midpoint of a line segment
from the coordinates of its end points. |
|
15 |
Algebraic representation and formulae |
·
Use letters to expressed generalised numbers and express basic
arithmetic processes algebraically;
·
Substitute numbers for words and letters in formulae;
·
Transform simple and more complicated formulae;
·
Construct equations from given situations. |
|
16 |
Algebraic manipulation |
·
Manipulate directed numbers;
·
Use brackets and extract common factors;
·
Expand products of algebraic expressions;
·
Factorise expressions of the form
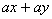 ; ; 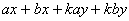 ; ; 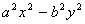 ; ; 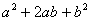 ; ; 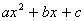 ; ;
·
Manipulate simple algebraic fractions. |
|
17 |
Indices |
·
Use and interpret positive, negative, zero and fractional indices. |
|
18 |
Solutions of equations and inequalities |
·
Solve simple linear equations in one unknown;
·
Solve fractional equations with numerical and linear algebraic
denominators;
·
Solve simultaneous linear equations in two unknowns;
·
Solve quadratic equations by factorisation and either by use of the
formula or completing the square;
·
Solve simple linear inequalities. |
|
19 |
Geometrical terms and relationships |
·
Use and interpret the geometrical terms: point, line, plane, parallel,
perpendicular, right angle, acute, obtuse and reflex angles, interior
and exterior angles, regular and irregular polygons, pentagons,
hexagons, octagons, decagons;
·
Use and interpret vocabulary of simple solid figures: cube, cuboid,
prism, cylinder, pyramid, cone, sphere;
·
Use the relationships between area of similar triangles, with
corresponding results of similar figures and extension to volumes of
similar solids. |
|
20 |
Geometrical constructions |
·
Measure lines and angles;
·
Construct simple geometrical figures from given data using protractors
or set squares as necessary;
·
Construct angle bisectors and perpendicular bisectors using straight
edges and compasses only;
·
Read and make scale drawings (Where it is necessary to construct a
triangle given the three sides, ruler and compasses only must be
used.) |
|
21 |
Bearings |
·
Interpret and use three-figure bearings measured clockwise from the
north (i.e.  ). ). |
|
22 |
Symmetry |
·
Recognise line and rotational symmetry (including order of rotational
symmetry) in two dimensions, and properties of triangles,
quadrilaterals and circles directly related to their symmetries;
·
Recognise symmetry properties of the prism (including cylinder) and
the pyramid (including cone);
·
Use the following symmetry properties of circles
(a)
Equal chords are equidistant from the centre;
(b)
The perpendicular bisector of a chord passes through the centre;
(c)
Tangents from an external point are equal in length. |
|
23 |
Angle |
·
Calculate unknown angles and solve problems (including problems
leading to some notion of proof) using the following geometrical
properties:
(a)
Angles on a straight line;
(b)
Angles at a point;
(c)
Vertically opposite angles;
(d)
Angles formed by parallel lines;
(e)
Angle properties of triangles and quadrilaterals;
(f)
Angle properties of polygon including angle sum;
(g)
Angle in a semi-circle;
(h)
Angle between tangent and radius of a circle;
(i)
Angle at the centre of the circle is twice the angle at the
circumference;
(j)
Angles in the same segment are equal;
(k)
Angles in opposite segments are supplementary. |
|
24 |
Locus |
·
Use the following loci and the method of intersecting loci:
(a)
Set of points in two dimensions
(i)
Which are at a given distance from a given point,
(ii)
Which are at a given distance from a given straight line,
(iii)
Which are equidistant from two given points;
(b)
Set of points in two dimensions which are equidistant from two given
intersecting straight lines. |
|
25 |
Mensuration |
·
Solve problems involving
(i)
The perimeter and area of a rectangle and a triangle,
(ii)
The circumference and area of a circle,
(iii)
The area of a parallelogram and a trapezium
(iv)
The surface area and volume of a cuboid, cylinder, prism, sphere,
pyramid and cone. (Formulae will be given for the sphere, pyramid and
cone.)
(v)
Arc length and sector area as fractions of the circumference and area
of the circle. |
|
26 |
Trigonometry |
·
Apply Pythagoras Theorem and the sine, cosine and tangent ratios for
acute angles to the calculation of a side or of an angle of a
right-angled triangle (angles will be quoted in, and answers required
in, degrees and decimals of a degree to one decimal place);
·
Solve trigonometrical problems in two dimensions including those
involving angles of elevation and depression and bearings;
·
Extend sine and cosine functions to angles between
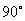 and and  ; ;
·
Solve problems using any sine and cosine rules for any triangle and
the formula 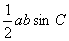 for the area of triangle; for the area of triangle;
·
Solve simple trigonometrical problems in three dimensions.
(Calculations of the angle between two planes or of the angle between
a straight line and a plane will not be required.) |
|
27 |
Statistics |
·
Collect, classify and tabulate statistical data;
·
Read, interpret and draw simple inferences from tables and statistical
diagrams;
·
Construct and use bar chars, pie charts, pictograms, dot diagrams,
stem-and-leaf diagrams, simple frequency distributions and frequency
polygons;
·
Use frequency density to construct and read histograms with equal and
unequal intervals;
·
Calculate the mean, median and mode for individual data and
distinguish between the purposes for which they are used;
·
Construct and use cumulative diagrams;
·
Estimate the median, percentiles, quartiles and interquartile range
from the cumulative frequency diagrams;
·
Calculate the mean for grouped data;
·
Identify the modal class from a group frequency distribution. |
|
28 |
Probability |
·
Calculate the probability of a single event as either a fraction or a
decimal (not a ratio);
·
Calculate the probability of simple combined events, using possibility
diagrams and tree diagrams where appropriate (in possibility diagrams
outcomes will be represented by points on a grid and in tree diagrams
outcomes will be written at the end of branches and probabilities at
the sides of the branches). |
|
29 |
Transformations |
·
Use the following transformations of the plane: reflection (M),
rotation (R), translation (T), enlargement (E), shear (H), stretch
(S), and their combinations (if M(a)=b and R(b)=c the notation RM(a)=c
will be used; invariants under these transformations may be assumed);
·
Identify and give precise descriptions of transformations connecting
given figures; |
|
30 |
Vectors in two dimensions |
|